Thermodynamic potentials

The four siblings: $u$, $h$, $f$, and $g$ (no others, no more allowed).

By construction of the Legendre transformations, each of these has units of energy. But what do they mean?

Read Chapter 8 (content starts now).

Internal energy - $u$

Our Gibbs law says, $$du=Tds - Pdv,$$ where $Tds=\delta q_r$.

So if the volume is not changing, $dv=0 \Rightarrow \color{blue}(du)_v=\delta q_r$.

One way of stating this:

For isochoric, reversible processes, no work is done on or by the system, and the heat flow is exactly equal to the change in internal energy, $du$.

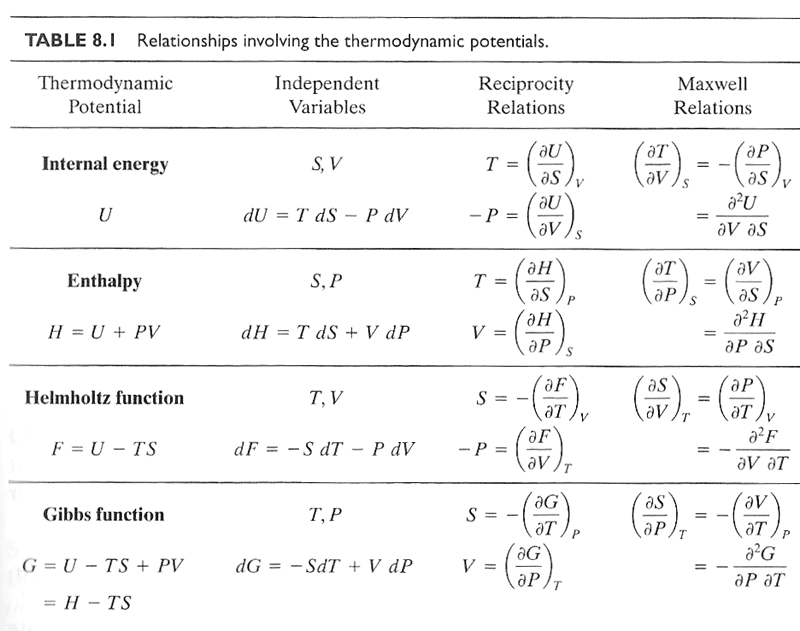
Maxwell relationships

Gibbs law is, $$du=Tds - Pdv.\label{GibbsLaw}$$

Hmmm, $s$ and $v$... We can in principal write $u$ as a function of any two independent thermodynamic parameters/function, for example$u=u(s,v)$.

With these variables, the Pfaffian of $du$ is: $$du = \left(\frac{\partial u}{\partial s} \right)_v ds + \left(\frac{\partial u}{\partial v} \right)_s dv,\label{Pfaffian}$$

Since we can equate ($\ref{GibbsLaw}$) and ($\ref{Pfaffian}$), and since $ds$ and $dv$ can vary independently, the coefficients of $ds$ and $dv$ must be equal, that is: $$\left(\frac{\partial u}{\partial s} \right)_v = T; \hspace{2.0em} \left( \frac{\partial u}{\partial v} \right)_s = -P.$$

Since $du$ is an exact differential, $\frac{\del^2 u}{\del s\del v}=\frac{\del^2 u}{\del v\del s}$, such that: $$ \left[ \frac{\partial T}{\partial v}\right]_s = \left[ -\frac{\partial P}{\partial s} \right]_v .$$

This is one of the so-called Maxwell relations. Three more exist for the other three thermodynamic potentials.

This is an example of what Kerson Huang wrote...

Enthalpy - $h$

Using the definition of enthalpy: $$h \equiv u + Pv \Rightarrow dh = du +Pdv +vdP.$$ The units of $h$ are energy, just like $u$.

Using Gibbs law $du = Tds -Pdv$ to substitute for $du$ in the expression above, we get: $$dh = Tds + v\,dP.\label{dh}$$ So, if $dP=0$, then $(dh)_P=(Tds)_P=(\delta q_r)_P$, or...

$dh$ is the heat flow for reversible processes when the pressure is not changing (isobaric processes).

Equation ($\ref{dh}$) suggests that we can think of $h=h(s,P)$ and $dh$ to its Pfaffian, $dh=(\del h/\del s)_P ds+(\del h/\del P)_s dP$ and another Maxwell relationship follows from this: $$ \left( \frac{\partial T}{\partial P}\right)_s = \left( \frac{\partial v}{\partial s} \right)_P .$$

Enthalpy, $h$, is the second of the four "thermodynamic potentials". It can be thought of as Legendre transformation of $u$:

Legendre transformation
Given a function of $x$ and $y$, $f(x,y)$, there exists a recipe: $$f(x,y)\pm\frac{\partial f}{\partial x}x\equiv g\left(\frac{\partial f}{\partial x},y\right)$$ which is guaranteed to produce a new function, $g$ (same units as $f$) which depends on the two independent variables $\frac{\partial f}{\partial x}$ and $y$.

Starting with Gibbs law... $du=T\,ds-P\,dV$, where $u=u(s,v)$, the correspondences are...

• $f\equiv u$,
• $x\equiv v$,
• $y\equiv s$,
• $ \frac{\del f}{\del x} \equiv \color{blue}{\frac{\del u}{\del v}=-P}$
• $g=f(x,y)-\frac{\del f}{\del x}\,x \equiv \color{blue}{h(s,P)=u+Pv} $

The Legendre transformations of internal energy, $u$

	$du=T\,ds - P\,dv$	$u(s,v)$	$\partial u/\partial v = -P$ $\partial u/\partial s = T$
$h=u+Pv$	$dh = T\,ds + v\,dP$	$h(s,P)$
$f = u - Ts$	$df = -s\,dT - P\,dv$	$f(T,v)$
$g = u -Ts+Pv$	$dg=-s\,dT+v\,dP$	$g(T,P)$

You can show any of these differentials by differentiating the definition of the function, and using $du=T\,ds-P\,dv$, then simplifying.

Helmholtz energy - $f$

$$f \equiv u - Ts.\label{eff}$$

The differential is: $$df = du-s\,dT - T\,ds.\label{deff}$$

Using Gibbs law $du= TdS -P\,dv$ in this,

$$\Rightarrow df = -s\,dT - P\,dv.$$ Making it look like $f=f(T,v)$ is the most natural form.

Constant $T$ process

Imagine a system in contact with a large, constant temperature reservoir. Under such conditions,

$$-[df]_T = P\,dv = \delta w_r.$$

So, the Helmholtz energy is apparently equal to (minus) the work done in a reversible, isothermal process.

Since a reversible process is the best we can do, we could say that

The Helmholtz energy, $f$ is the maximum mechanical energy to be had from an isothermal process.

In German, work is "Arbeit", and so some texts have used $a(T,v)\equiv f(T,v)$--a convention that the IUPAC (International Union of Pure and Applied Chemistry) wants to adopt, but it hasn't caught on yet. They also resolved (1988) to banish the previous term "free energy", yeah, that's where $f$ apparently comes from :-<.

Constant $T$, constant $v$ process

We shall see that...

Pragmatically, $f$ is useful to find the equilibrium state when temperature and volume are held constant.

Since, $$df = -s\,dT - P\,dv.$$ for a process at constant $T$ and constant $v$, it looks like $[df]_{T,v}=0$ once equilibrium has been reached. But is $f$ a maximum or a minimum here?

Now, let's redefine "the system" to consist of a small system + a large heat reservoir:

• Our original, now small system, is still inside of a rigid container ($dv_s=0$, where the $s$ now means the small system,
• Our small system is in diathermal contact with a larger system, a heat reservoir.
• Heat can flow back and forth between the two subsystems.
• The large reservoir is at a constant temperature, $T$.
• But the reservoir is large enough that when heat flows in and out, its temperature does not change $\Rightarrow dT_r=0$,
• And, since its temperature is not changing, it's volume is also unchanging $\Rightarrow dV_r=0$.
• Since $dF = -s\,dT-P\,dV$, this means that even if heat flows in and out of the reservoir, $\color{blue}dF_r = 0$!

So, heat may flow in or out of the small system, and the net change in the Helmholz energy of this compound system is $$dF=dF_s+dF_r= dF_s.$$ Now, in any spontaneous process, the net entropy of the total system = small system + the reservoir must increase: $$dS_s + dS_r \geq 0.$$ and according to the second law $$dS = \frac{\delta Q_r}{T} \geq \frac{\delta Q}{T}.$$ (Conveniently, the $r$ of reversible in this expression works also as the $r$ in reservoir, which can reversibly absorb or give off heat!)

So we're consider a joint system of small system in contact with a reservoir at temperature $T$. Imagine that this joint system is isolated from their surroundings.

If the reservoir transfers some heat $\delta Q$ to the system, then $dS_{res} = -\delta Q_s/T$. Subbing this in and re-arranging... $$T dS_r \geq \delta Q_s.$$

Or, re-arranging, and dividing by $n$ for the system... $$0 \geq \delta q_s - Tds.\label{2ndLawConsequence}$$

Returning to...

• the Helmholtz energy, Eq ($\ref{eff}$), $f\equiv u-Ts$,
• its differential, Eq ($\ref{deff}$) $df = du-Tds-s\,dT$

This time, let's substitute the first law written as, $du = \delta q - Pdv$ into the differential $df$: $$\begineq df&=\delta q-Pdv-Tds-s\,dT\\ &= (\delta q-Tds)-Pdv-s\,dT \endeq$$

For a constant volume, constant temperature process, the last two terms vanish, leaving... $$\left[df\right]_{v,T}=\delta q -Tds \leq 0$$

The inequality, from Eq ($\ref{2ndLawConsequence}$, is a consequence of the second law. This means that $f$ can spontaneously decrease (but not increase) when $v$, and $T$ are held constant. The conclusion is:

If a system is being held at a constant volume and a constant temperature, but is not initially at equilibrium, it may change spontaneously to a state in which $f$ is lower. When the system is no longer changing, its Helmholtz energy, $f$, is at a minimum, such that $\left[df\right]_{v,T}=0$. This defines the equilibrium state. (isochoric, isothermal system).

We will soon use this to find the precise phases for a van der Waals gas below its critical temperature

Gibbs energy - $g$

The Gibbs energy (or sometimes Gibbs free energy) is, $$g = u -Ts+Pv.$$

Once more using $du=\delta q-P\,dv$, we can write the differential as $$\begineq dg &= du -T\,ds-s\,dT+P\,dv+v\,dP\\ &= \delta q-Pdv -Tds-s\,dT+Pdv+v\,dP\\ &= (\delta q -Tds)-s\,dT+v\,dP \endeq$$

Arguing as before, if $T$ and $P$ are held constant, $$\left[ dg \right]_{T,P} \leq 0.$$

A system constrained to have a constant temperature and constant pressure can spontaneously move to lower $g$, and an equilibrium system is sitting at a minimum of the the Gibbs energy $g$.

AT LAST: these are the variables we can most easily control in the lab: Typically we hold $P$ constant by doing chemistry in an open container, such that the pressure remains at atmospheric pressure, despite possible changes in volume.),

We will soon use this for chemistry: To find things like the equilibrium concentrations of reactants and products in chemical reactions.

Relation to the other thermodynamic potentials... $$\begin{align}g & = u - Ts +Pv\\ &= f + Pv = h - Ts.\end{align}$$

Canonically conjugate variables

Instead of transforming the dependence of the internal energy dependence on $v \rightarrow P$, we could find Legendre transformations of $u$ that would depend on $T$ and $v$, or on $T$ and $P$ instead.

The resulting functions would have differentials that depend on combinations like $T\,ds$, or $s\,dT$, or $P\,dv$, or $v\,dP$, but never combinations like $T\,dP$. We give this kind of relationship a special name:

• $T$ and $S$ are canonically conjugate variables (heat-related).
• $P$ and $V$ are canonically conjugate variables (mechanical).

Notice that in each pair there is one quantity that is only ever intensive ($T$ and $P$), and one extensive quantity ($S$ and $V$).

We can extend our equations to include non-mechanical forms of work. For example, in an electric circuit, there is work associated with moving a chunk of charge $dQ_e$ (*not* a heat--sorry!) through a voltage difference ${\mathcal E}$: $$\delta W = {\mathcal E} dQ_e.$$

• $Q_e$ and ${\mathcal E}$ are canonically conjugate,
• the amount of charge $Q_e$ is extensive, and
• the voltage difference ${\mathcal E}$ is intensive.

Summary

Image credits

Esther Gibbons