Connection between classical and statistical thermodynamics
Work?....Heat?....
How should we understand these classical terms in statistical mechanics?
$$U=\sum_jN_jE_j.$$
So, $$dU = \sum_jE_jdN_j + \sum_jN_jdE_j.$$
Because $E_j = E_j(V)$, $\Rightarrow dE_j = (dE_j/dV)dV$, so the second term becomes... $$\sum_jN_jdE_j = \sum_j \left(N_j\frac{dE_j}{dV}\right)dV= - \sum_j Y\,dV.$$ That is, $Y=-\sum_j \left(N_j\frac{dE_j}{dV}\right)$. [The negative sign is a reminder that with energy levels of the particle in a box, $E_j \propto n_j^2/V^{2/3}$, as $V$ increases, the energy of level $j$ drops.]
So, in the statistical picture, we have: $$dU = \sum_j E_j\,dN_j - Y\,dV.$$
Classically: $$dU = T\,dS - P\,dV.$$
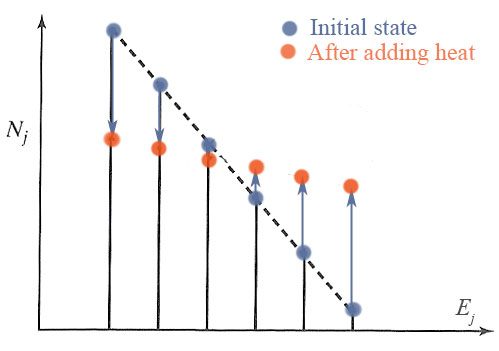
Holding $V$ constant, we have in the classical case, followed by the statistical case: $$\left(dU\right)_V = T\,dS = \delta Q_r = \sum_j E_j\,dN_j .$$
Apparently heating is associated with a change of the number of particles in energy levels, while keeping the energies of each level constant.
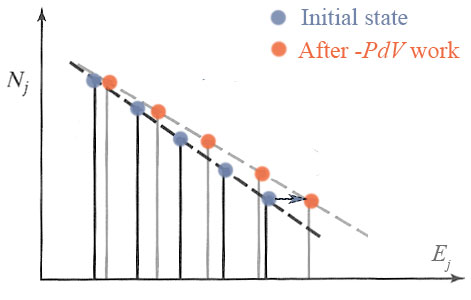
The remainder, $$dU - \delta Q_r = -\delta W_r = -Y\,dV = \sum_j N_j \,dE_j.$$
Particle in a box: $E_j \propto n_j^2/V^{2/3}$.
So, squeezing the box smaller increases the spacing between levels, and moves them all up.
Apparently work done *on* the system is associated with a shift in the energy levels (while keeping the number of particles in each state constant.)