Induced electric fields
...an "Ampere's Law" for the electric field.
Faraday's law can be written as: $${\cal E} = \oint_{\cal P} \myv E \cdot d \myv l = -\frac{d}{dt}\int_{\cal S} \myv B \cdot d \myv a.$$
The path integral around a closed path can be written, according to Stoke's theorem, as a surface integral of a cross product, so: $$\int_{\cal S} \myv \grad \times \myv E \cdot d \myv a = -\frac{d}{dt}\int_{\cal S} \myv B \cdot d \myv a.$$
If the magnetic field is changing, but not the loop (and thus the surface), the differential can be passed under the integral like... $$\int_{\cal S} \myv \grad \times \myv E \cdot d \myv a = -\int_{\cal S} \frac{\del \myv B}{\del t} \cdot d \myv a.$$
To be true for all fixed surfaces, it has to be the case that $$\myv \grad \times \myv E = - \frac{\del \myv B}{\del t}.$$
With magnetic fields, this was the sequence of things that lead to Ampere's law: $$\myv \grad \times \myv B = \mu_0 \myv J$$ $$ \text{and } \myv \grad \cdot \myv B = 0$$ $$\Rightarrow \oint \myv B \cdot d \myv l = \int \mu_0 \myv J\cdot d\myv a=\mu_0 I_\text{enc}.$$
Now with induced fields, as long as there's no charge density around, $\rho=0\propto\myv \grad \cdot \myv E$. So we can write... $$\myv \grad \times \myv E = -\frac{d}{dt}\myv B$$ $$\myv \grad \cdot \myv E = 0]$$ $$\Rightarrow \oint \myv E \cdot d \myv l =\int\(-\frac{d}{dt}\myv B\)\cdot d\myv a= -\frac{d}{dt} \Phi_B.$$
It looks like $-\frac{d}{dt} \Phi_B$ plays the same role as $I_\text{enc}$ played in magnetostatics. So we can use our whole, symmetry-related "bag of tricks" from Ampere's law now on electric fields where the change of flux plays the role of the enclosed current. For example...
Example: An infinitely long straight wire carries a slowly varying current $I(t)$. What is the induced electric field near the wire? First off...what direction is the electric field?
Try putting up Amperian loops where the surface area is perpendicular to... $\uv s$, $\uv z$, and $\uv{\phi}$ in turn... In which geometry is there a changing $B$-flux through the loop?
Convince yourself that it's only when the loop is perpendicular to $\uv{\phi}$
that there's a change in the flux.
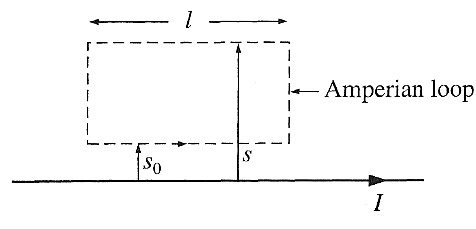
Then, we've got: $$\oint \myv E \cdot d\myv{l} = E_z(s_0)l - E_z(s)l = -\frac{d}{dt}\int \myv B \cdot d \myv a,$$
eventually... $$\Rightarrow \myv E(s) = \left(\frac{\mu_0}{2\pi} \frac{dI}{dt} \ln s + C\right) \uv{z}.$$
Inductance
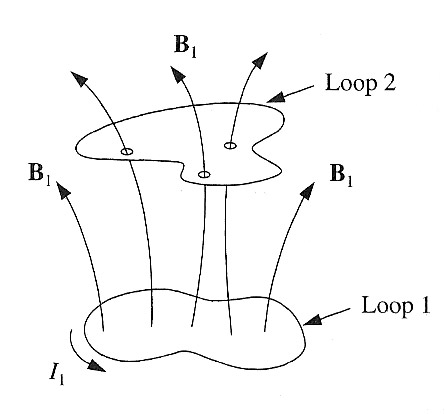 A current
$I_1$ flows in loop 1, giving rise to a magnetic field $\myv B_1$. What's the
flux $\Phi_2$ through loop 2 of the field $\myv B_1$?
$$\Phi_2 = \int \myv B_1 \cdot d\myv{a}_2.$$
A current
$I_1$ flows in loop 1, giving rise to a magnetic field $\myv B_1$. What's the
flux $\Phi_2$ through loop 2 of the field $\myv B_1$?
$$\Phi_2 = \int \myv B_1 \cdot d\myv{a}_2.$$
We can in principle calculate $\myv B_1$ through the B-S law: $$\myv B_1 = \frac{\mu_0}{4 \pi}I_1 \oint \frac{d\myv{l}_1 \times \myv \rr}{\rr^2}.$$
Without actually solving this integral, we can see that $\myv B_1 \propto I_1$ which in turn implies $\Phi_2 \propto I_1$, that is.. $$\Phi_2 = M_{21}I_1,$$ where the constant of proportionality $M_{21}$ is called the mutual inductance. (Units: "henries" (H), where 1 H = 1 volt-second / ampere).
Developing this further by means of the magnetic vector potential... $$\begineq \Phi_2 &= \int \myv B_1 \cdot d\myv{a}_2 = \int \left(\myv \grad \times \myv A_1\right)\cdot d \myv a_2\\ &= \oint \myv A_1 \cdot d\myv l_2.\endeq$$
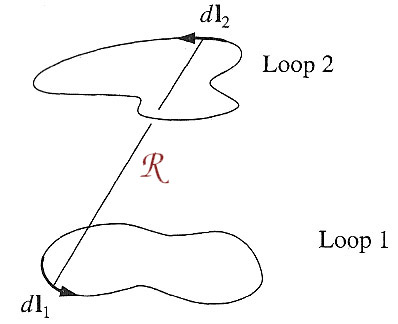 We've seen the general expression for the magnetic vector potential:
$$\myv A_1 = \frac{\mu_0 I_1}{4 \pi} \oint \frac{d \myv l_1}{\rr}.$$
We've seen the general expression for the magnetic vector potential:
$$\myv A_1 = \frac{\mu_0 I_1}{4 \pi} \oint \frac{d \myv l_1}{\rr}.$$
Subbing... $$\begineq\Phi_2 &= \frac{\mu_0 I_1}{4 \pi}\oint \left(\oint \frac{d\myv l_1}{\rr}\right)\cdot d\myv{l}_2\\ &= \left[\frac{\mu_0}{4 \pi}\oint \oint \frac{d\myv{l}_1 \cdot d\myv{l}_2}{\rr}\right] I_1.\endeq$$
This is the Neumann formula. The quantity in square brackets $[..]=M_{21}=M_{12}\equiv M$. Apparently the mutual inductance only depends on the geometric arrangement of the current loops, and not the field.
Now, just as the flux in a second loop is proportional to the current in the first loop, there is also a dependence of the flux through one loop, on the current in that same one loop itself: $$\Phi = L I,$$ where $L$ is the self-inductance.
An ${\cal E}$ (a 'back-${\cal E}$') will be induced by any change in the flux, which is (for rigid loops)... $${\cal E} = -\frac{d}{dt}\Phi = -L \frac{dI}{dt}.$$
We shall shortly see that $L$ acts like mass in an electrical circuit, lending the circuit some "inertia".
L-R circuit
Every circuit, whether it has an inductor in it or not, is a current loop and so has some self inductance $L$. 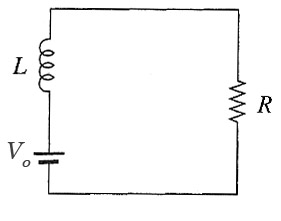 This self inductance is pictured as an additional circuit element here, but it's inherent in the circuit.
This self inductance is pictured as an additional circuit element here, but it's inherent in the circuit.
If current is flowing already, unplugging (or suddenly cutting) the circuit causes a very rapid $\frac{dI}{dt}$, and a spark is often generated.
What about when you suddenly plug a circuit in? $I(t=0) = 0$.
We'll have $$V_0 + {\cal E} = V_0-L\frac{dI(t)}{dt} = I(t)R.$$
To solve this, re-write it as... $$-\frac{L}{R}\frac{dI(t)}{dt} = I(t)-\frac{V_0}{R}.$$
Now define $F(t) \equiv I(t)-\frac{V_0}{R}$, and note that $\frac{dF}{dt} = \frac{dI}{dt}$, so we can re-write this equation as... $$-\frac{L}{R}\frac{dF(t)}{dt} = F(t).$$
The solution is an exponential... $$F(t) = ke^{(-R/L)t}=I(t)-V_0/R,$$
And so we conclude... $$\Rightarrow I(t)= ke^{(-R/L)t} + V_0 /R.$$
If $I(t=0)=0$, then $k=-V_0 /R$, so... $$I(t)=\frac{V_0}{R}(1-e^{(-R/L)t}).$$
Magnetic energy
 Wardenclyffe
Tower was Nicola Tesla's attempt to transmit energy and messages across the Atlantic from Long
Island to Europe.
Wardenclyffe
Tower was Nicola Tesla's attempt to transmit energy and messages across the Atlantic from Long
Island to Europe.
Just as we found that there is an energy $W=\frac{1}{2}\int_{\cal V}\myv D\cdot \myv E\,d\tau$ associated with an electric, field, I want to show that there is also an energy that can be calculated from the magnetic field.
If some sort of voltage source (battery) $V_0$ is applied to a circuit, as above in general we have $$V_0 + {\cal E} = IR.$$
The work done by the battery in moving a small chunk of charge $dq=I\,dt$ once around the circuit is $$\begineq dW &= V_0 dq = V_0Idt=-{\cal E}Idt+I^2Rdt\\ &=Id\Phi + I^2R\,dt.\endeq$$
That last term is apparently Joule heating, and represents energy irreversibly lost to heat.
The first term is work that is done to set up the magnetic fields, and can be reversibly extracted from the system.
For a single, rigid loop circuit, $\Phi = LI \Rightarrow d\Phi = LdI$. So the work done to change the magnetic state of things $dW_B$ is $$dW_B=LI\,dI.$$
We can integrate this to get the total work done, starting from zero current... $$\begineq W_B &= \int_0^ILI'dI' \\ &= \frac{1}{2}LI^2 = \frac{1}{2}\Phi I=\frac{1}{2}\Phi^2 /L.\endeq$$
..in terms of magnetic fields
$$\Phi = \int_{\cal S} \myv B\cdot d\myv a = \int_{\cal S} (\myv \grad \times \myv A)\cdot d\myv a = \oint_{\cal C} \myv A\cdot d\myv{l}.$$We can substitute this in to our work expression $$W_B=\frac{1}{2}\oint_{\cal C} I \myv A\cdot d\myv{l}.$$
It would be nice to write this in terms of current density instead of total current. Substituting $I\,d\myv{l} \to \myv J\,d \tau$ and then $\oint_{\cal C} \to \int_{\cal V}$... $$W_B =\frac{1}{2} \int_{\cal V} \myv A \cdot \myv J d \tau =\frac{1}{2\mu_0} \int_{\cal V} \myv A \cdot (\myv \grad \times \myv B) d \tau.$$
Pulling out handy vector identity #6, we can re-write... $$\myv A\cdot (\myv \grad \times \myv B) = \myv B \cdot \myv B - \myv \grad \cdot (\myv A \times \myv B).$$
Using this in the energy expression:
$$\begineq W_B &=\frac{1}{2\mu_0} \left[\int_{\cal V} B^2 d \tau -\int \myv \grad \cdot
(\myv A \times \myv B) d \tau\right]\\
&= \frac{1}{2\mu_0} \left[\int_{\cal V} B^2 d \tau -\oint_{\cal S} (\myv A \times \myv B ) \cdot d\myv{a}\right].\endeq$$
Take ${\cal V}$ and ${\cal S}$ to be over all space--Why not, since we'll for sure catch the volume where the source currents are non-zero that way. Some things to note about the $\oint_{\cal S}$ integral
- $\myv A \propto 1/r,$
- $\myv B \propto 1/r^2,$
- $d \myv a \propto r^2.$
So, as $r\to \infty$, that second integral is dropping off as $1/r \to 0$. So we can neglect it compared to the first integral...
$$W_B = \frac{1}{2\mu_0}\int_{\cal V} B^2 d \tau.$$