Entropy of mixing

Consider two gases, at the same temperature and pressure, but initially separated by a removable wall. After the wall is removed, the two gases diffuse around, and eventually we end up with a homogeneous mixture.

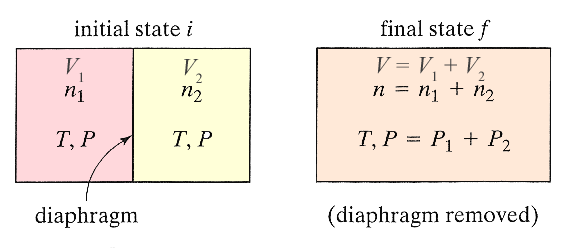
Mixing is an irreversible process, so there ought to be an associated increase in entropy. How much?

In general, the chemical potential of each type of stuff in the system depends on its environment. So it depends on the concentration of the other stuff around it. We'd like to not worry about this sort of concentration dependence, so to simplify further, let's assume these two gases are both ideal:

Simplifying to the case of ideal gases

We noted that ideal gases behave as if they were made of molecules that have neither a positive nor a negative interaction energy with others of their kind.

It seems like not too much of a stretch to believe that if the two gases we mix are both ideal, that the molecules of one will also have no interaction energy (either positive or negative) with the molecules of the other type.

Too simple? If this turns out to be too much of a simplification, we will be left with no entropy change whatsoever upon mixing. Entropy will turn out to have something to do with the energies of interaction of different kinds of matter.

But if this is *not* too much of an oversimplification, we should see a change of entropy, and this will mean that an entropy change depends in some very pure sense on how the molecules are arranged in the container, and not on the energetics of their interactions.

Let's see!

Isothermal expansions

Since we're going to ignore the interactions with other gases, we could do the expansion like this, expanding gas 1 first, and then gas 2. (Since entropy is a state function...

Imagine that we have two special membranes that we can move separately, each membrane is permeable to only one kind of matter, but not the other. They start at the same position in the container (see image) with gas 1 on the left and gas 2 on the right.

In the first step, we expand gas 1 from $V_1$ to $V=V_1+V_2$ by moving the membrane that is permeable to gas 2 (but not gas 1) to the right. Since $U(T,V)=U(T)$, the internal energy does not change, and the temperature is constant. Now, considering as our system only molecules of type 1... $$dU=0=T\,dS -P\,dV.$$

Re-arranging for $S$ and integrating $$\begin{align}\Delta S_1 & = \int dS = \int\frac{P}{T}dV=n_1R\int\frac{dV}{V} \\& =n_1R \ln(V/V_1)\end{align}.$$

Or, since

• $V_1 = n_1RT/P$, and
• $V=V_1+V_2 = (n_1+n_2)RT/P$, and
• the total number of moles is $n_1+n_2 = n$, and
• the final mole fraction (concentration) is $x_1=n_1/n$,

$$\Delta S_1 = n_1R\,\ln(n/n_1)=-n_1R\, \ln x_1.$$

Similarly, when we move the second membrane to the left, we find $$\Delta S_2 = -n_2R\, \ln x_2=-nR\left( \frac{n_2}{n}\ln x_2\right)=-nRx_2\,\ln x_2.$$

The total change of entropy is... $$\Delta S = -nR(x_1\,\ln x_1 + x_2\,\ln x_2).$$

To check... what if the partition is all the way at the left, and the mole fractions of the two gases are $x_1 = 0, x_2=1$? You should find $\Delta S=0$.

Since $x_2=1-x_1$, we can graph $\Delta S(x_1)$:

The maximum possible change in entropy occurs when $x_1 = x_2=1/2$: $$\Delta S = -nR(2\cdot \frac{1}{2}\ln(1/2)) = nR\,\ln2.$$

So, in the case of ideal gases, it appears that there *is* an entropy increase on mixing, and it has something to do with the greater volume available for each molecule.

It is not difficult to generalize this result to mixing of many ideal gases... $$\Delta S = -nR\sum_{i} x_i\,\ln x_i.$$

Gibbs paradox

But a striking thing occurs when we pull out a wall separating equal amounts of the same ideal gas. It would appear at first blush that the entropy change should still be: $$\Delta S = nR\, \ln 2.$$

How is this possible? There shouldn't be a change in entropy if the gases on both sides of the partition are the same...should there?

Can you see any weakness(es) in the arguments leading up to the expression above if we were mixing identical gases? What do you think is the correct expression for mixing two quantities of the same gas?